Circular Motion.
Whenever an object goes round in a circle it does so because of a force thatis causing it. The net resultant force towards the centre is the centripetal force.
----------------------------------------------------------------
An introduction to circular motion and why acceleration is towards the centre of the circle. (Click on the diagram to link to the external sites)
.PNG)
----------------------------------------------------------------
Looking closer at the maths..PNG)
----------------------------------------------------------------
This can be summarised in the following diagram.
We can also look at the case of motion in a verticle circle. The cetripedal force is the resultant force. The other forces combine to cause this and are the contact froce of the chair (or tension in the string in the second example) and the weight (F=mg)of the object.
and again
.PNG)
----------------------------------------------------------------
On a carousel the forces combine to cause and make up the centripetal acceleration.
----------------------------------------------------------------
The following applet shows how circular motion is closly linked to sine waves and SHM.
.PNG)
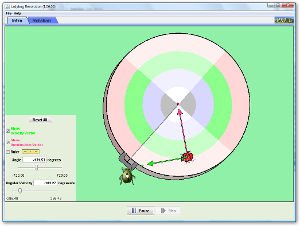
The following applet can be downloaded to further investigate circular motion. Join the ladybug in an exploration of rotational motion. Rotate the merry-go-round to change its angle, or choose a constant angular velocity or angular acceleration. Explore how circular motion relates to the bug's x,y position, velocity, and acceleration using vectors or graphs
| Click to Run |
----------------------------------------------------------------
----------------------------------------------------------------
----------------------------------------------------------------
The following is a presentation that has been used in class with A'level pupils. Open it in a new tab.
----------------------------------------------------------------
UNDER CONSTRUCTION